Question

Find the zeroes of the function and write in a+bi form. -(x+1)^2-4=0

Answer 1

x = 2 + √-16 [ (-2)⁻¹]

See steps below.

Explanation:

Finding the zeroes of a function simply means solving for the unknown variable (in this case "x") when the function is equated to zero.

The function here is
`f(x) = -(x + 1)^(2) - 4 = 0`

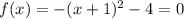
Start by opening the bracket. Ignore the minus sign. It will be reintroduced at the end of this step.

(x + 1)(x + 1) = x² + x + x + 1 = x² + 2x + 1

Reintroduce the minus sign. Don't forget to put the result above back in a bracket.

- (x² + 2x + 1) = -x² -2x -1

Place the expression back in the equation and solve. Make sure to put it back in the position where it was.

-x² - 2x - 1 - 4 = 0

Simplify the non-algebraic terms.

-x² - 2x - 5 = 0

Use Quadratic Formula to solve for x.

Quadratic formula: x = -b ± √(b² - 4ac)

2a

a = the coefficient of x² = -1

b = the coefficient of x = -2

c = the unit term = -5

So x = -(-2) ± √ [(-2)² - 4(-1 x -5)]

2(-1)

x = 2 + √-16 or x = 2 - √-16

-2 -2

This is where the evaluation ends, since the square root of minus sixteen cannot be evaluated. x hence is equal to a complex number. The above are the two values for x.

Next step is to put it in "a + bi" form.

x = 2 + √-16 ÷ -2 = 2 + √-16 x (-2)⁻¹

So x = 2 + √-16 [ (-2)⁻¹]

where

a is 2;

b is √-16

i is [(-2)⁻¹]