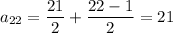The given sequence is arithmetic with a common difference of

between each term.
Recursively, you can define the sequence as

and solve explicitly for the

th term by recursively substituting the right hand side:

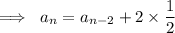
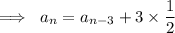
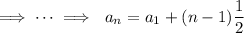
The first term of the sequence is
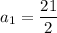
, so the

th term is given by the formula
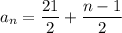
So, the 22nd term in the sequence is