Question

The surface area of a sphere is decreasing at the constant rate of 3*pi sq. cm/sec . ? At what rate is the volume of the sphere decreasing at the instant its radius is 2 cm ?

Answer 1

Surface area of a sphere:
`A=4\pi r^2`
Volume of a sphere:
`V=\frac43\pi r^3`

Differentiate both with respect to an arbitrary variable for time:

`\displaystyle(\mathrm dA)/(\mathrm dt)=8\pi r(\mathrm dr)/(\mathrm dt)`

`\displaystyle(\mathrm dV)/(\mathrm dt)=4\pi r^2(\mathrm dr)/(\mathrm dt)`

You're given that
`(\mathrm dA)/(\mathrm dt)=-3\pi` when
`r=2`, so you can use this in the first equation to solve for
`(\mathrm dr)/(\mathrm dt)`.


`-3\pi=8\pi *2(\mathrm dr)/(\mathrm dt)\implies(\mathrm dr)/(\mathrm dt)=-\frac3{16}`

Now use this to find
`(\mathrm dV)/(\mathrm dt)`.


`\displaystyle(\mathrm dV)/(\mathrm dt)=4\pi *2^2*\left(-\frac3{16}\right)=-3\pi`

Answer 2

9.42cm^3/s

Explanation:

The surface area of a sphere is decreasing at the constant rate of 3*pi sq. cm/sec . ? At what rate is the volume of the sphere decreasing at the instant its radius is 2 cm ?

We know a sphere has a volume and area

V=4/3
`\pi r^3 and A=4\pi r^2`

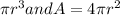
dA/dt=dA/dr*dr/dt............1

also dV/dt=dV/dr*dr/dt... ........

from equation 1

we can make dr/dt the subject of the equation

dr/dt=dA/dt/(dA/dr)

substitute into equation 2

dV/dt=dV/dr*(dA/dt/(dA/dr))

dv/dr=
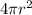`4\pi r^2`=4*3.14*2^2=50.24

dA/dt= 3*pi

dA/dr=8*p1*2=

3/16*50.24

dV/dt=9.42cm^3/s

is the rate at which the volume is decreasing