Question

Are the following equations parallel,

perpendicular or neither?
y=-1/2+3 10x-5y=15

Answer 1

10x-5y=15
-5y = 15 - 10x
-5y = -10x + 15
5y = 10x - 15
y = 2x - 15

They are perpendicular as the negative reciprocal of 2 is -1/2

Answer 2

The given equations are perpendicular as their slopes are negative reciprocals.

Explanation:

Given equations:

`\begin{cases}y=-(1)/(2)x+3\\\\10x-5y=15\end{cases}`

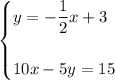
Rearrange the second question to isolate y:

`\implies 10x-5y=15`

`\implies 10x-5y+5y=15+5y`

`\implies 10x=15+5y`

`\implies 10x-15=15+5y-15`

`\implies 10x-15=5y`

`\implies 5y= 10x-15`

`\implies (5y)/(5)=(10x)/(5)-(15)/(5)`

`\implies y=2x-3`

`\boxed{\begin{minipage}{6.3 cm}\underline{Slope-intercept form of a linear equation}\\\\$y=mx+b$\\\\where:\\ \phantom{ww}$\bullet$ $m$ is the slope. \\ \phantom{ww}$\bullet$ $b$ is the $y$-intercept.\\\end{minipage}}`

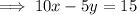
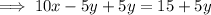
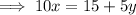
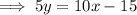
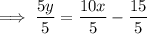
Therefore:

• The slope of the first equation is -¹/₂.
• The slope of the second equation is 2.

The slopes of parallel lines are the same.

The slopes of perpendicular lines are negative reciprocals.

The reciprocal of a number is 1 divided by the number.

Therefore, the negative reciprocal of 2 is -¹/₂.

The given equations are perpendicular as their slopes are negative reciprocals.