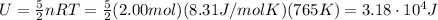Question

What is the internal energy of 2.00 mol of an ideal diatomic gas at 765 k , assuming all degrees of freedom are active?

Answer 1

The internal energy of an ideal gas is given by:

`U= (k)/(2)nRT`
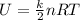
where
k is the number of degrees of freedom of the molecules of the gas
n is the number of moles
R is the gas constant
T is the absolute temperature.

For a diatomic gas, k=5. In our problem, the number of moles is n=2.00 and the absolute temperature of the gas is T=765 K, so its internal energy is

`U= (5)/(2)nRT= (5)/(2)(2.00 mol)(8.31 J/mol K)(765 K)=3.18 \cdot 10^4 J`