Question

1 3/17 + 1 3/17 + 9/17

`1 (3)/(17) + 1(3)/(17) + (9)/(17)`

Answer 1

The first step to solving this is to convert the first mixed number into an improper fraction.

`(20)/(17)` + 1
`(3)/(17)` +
`(9)/(17)`
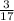
Now convert the second mixed number into an improper fraction

`(20)/(17)` +
`(20)/(17)` +
`(9)/(17)`
Since the denominator of each of these fractions are the same,, simply add each of the numerators of the fractions together in order to get your final answer.

`(49)/(17)`
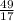
This means that the correct answer to your expression is
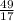
`(49)/(17)`,, or 2
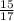
`(15)/(17)` simplified.
Let me know if you have any further questions
:)

Answer 2

`1(3)/(17)+1(3)/(17)+(9)/(17)=(20)/(17)+(20)/(17)+(9)/(17)=(49)/(17)=2(15)/(17)\approx2.88235.`