0.737373...=0.73+0.73(0.01)+0.73(0.01)²+0.73(0.01)³...
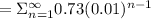
The sum is 73/99.
We start out with
0.737373... = 0.73 + 0.0073 + 0.000073 + 0.00000073 + ...
This is the same as
0.737373... = 0.73 + 0.73(0.01) + 0.73(0.0001) + 0.73(0.000001) + ...
Which is the same as
0.737373... = 0.73 + 0.73(0.01) + 0.73(0.01)² + 0.73(0.01)³ + ...
The term number, n, relates to the exponent; when n = 2, the exponent is 1; when n = 3, the exponent is 2; etc. This means the exponent is represented by n-1 in our series.
To find the sum, we see first that it is convergent because r = 0.01 < 1. We can find the sum using
a/(1-r), where a is the first term, 0.73:
0.73/(1-0.01) = 0.73/0.99 = 73/99