Answer:
Explanation:
Given that Chocolate chip cookies have a distribution that is approximately normal with a mean of 24.1 chocolate chips per cookie and a standard deviation of 2.1 chocolate chips per cookie
i.e. if X represents the number of chocolate chip cookies, we have X is N(24.1,2.1)
Convert to Z score
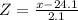
95% upper Z = 1.645 and lower is -1.645
Hence upper p 95 is

Lower p 5 would be
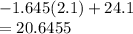
These two values give us an idea that the chocolate cookies should be within these two values.