The question is a bit of a mess, so here's an attempt at parsing out the relevant information.
Given the PDF of a random variable
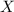
:
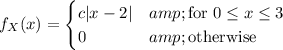
Find

, the CDF, the median of
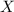
, and the expectation of
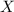
(in no particular order).
For
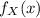
to be valid PDF, we require

Note that
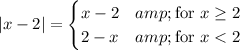
We have
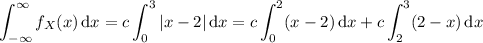
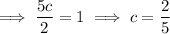
The CDF is defined by
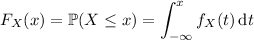
To find the CDF, we compute the integral above for the four possible cases:
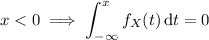
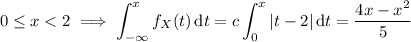
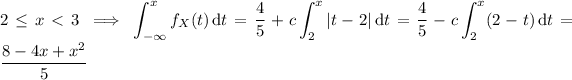
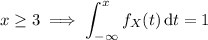
So the CDF is
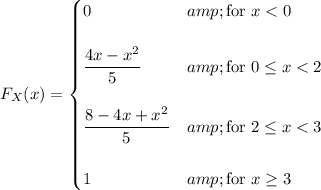
The median is the value
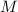
such that
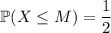
. From the PDF, we can gather that the median must fall somewhere in

. The CDF then tells us that

The expected value of
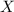
is given by
![\mathbb E[X]=\displaystyle\int_(-\infty)^\infty x\,f_X(x)\,\mathrm dx](https://img.qammunity.org/2019/formulas/mathematics/college/csgpk6127nnre60lv2wq7tf7660ffpkcph.png)
We have
![\mathbb E[X]=\displaystyle\frac25\int_0^2x(x-2)\,\mathrm dx+\frac25\int_2^3x(2-x)\,\mathrm dx=(16)/(15)](https://img.qammunity.org/2019/formulas/mathematics/college/ubfmdk588nj1nr5eens6phzi9oxsnqhk1e.png)