Answer:
The solution is

Explanation:
A compound inequality is an inequality that combines two simple inequalities.
We want to solve for x the following compound inequality

Solving the first inequality for x, we get:
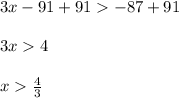
Solving the second inequality for x, we get:
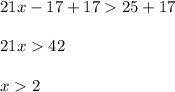
So our compound inequality can be expressed as the simple inequality:

The graph of a compound inequality with an "or" represents the union of the graphs of the inequalities. A number is a solution to the compound inequality if the number is a solution to at least one of the inequalities.
Graphically, we get