Question

Angles X and Y are supplementary. Angle X is 3 times the measure of angle Y. What is the measure of angle X?

Answer 1

supplementary angles sum = 180
lets x = measure of angle Y

x + 3x = 180
4x = 180
x = 45

angle Y = 45
angle X = 3 x 45 = 135

answer
Angle X = 135

Answer 2

The measure of ∠X is 135°

Explanation:

Given that angles X and Y are supplementary and also angle X is 3 times the measure of angle Y.

we have to find the measure of angle X.

Since X and Y are the supplementary therefore these add up to 180° i.e

`\angle X+\angle Y=180\°......(1)`

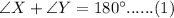
Also angle X is 3 times the measure of angle Y i.e

`\angle X=3\angle Y`

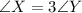
Substitute this value in equation (1), we get

`3\angle Y+\angle Y=180\°......(1)`

`4\angle Y= 180\°`

`\angle Y=(180\°)/(4)=45\°`

`\angle X=3* 45\°=135\°`

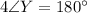
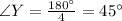
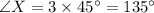
Hence, the measure of ∠X is 135°