Answer:
There are 210 ways in which you can pick 4 students from 10 students (6 men, 4 women).
Explanation:
We use the combination formula because the order does not matter.
For example, John, Laura,... is the same way as Laura, then John, then ...
Combinations formula
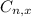 is the number of different combinatios of x objects from a set of n elements, given by the following formula.
is the number of different combinatios of x objects from a set of n elements, given by the following formula.
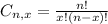
In this problem, we have that:
Combinations of 4 students from a set of 10. So
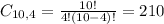
There are 210 ways in which you can pick 4 students from 10 students (6 men, 4 women).