To take out terms outside the radical we need to divide the power of the term by the index of the radical; the quotient will be the power of the term outside the radical, and the remainder will be the power of the term inside the radical.
First, lets factor 8:
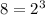
Now we can divide the power of the term, 3, by the index of the radical 2:
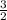
= 1 with a remainder of 1
Next, lets do the same for our second term
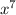
:
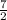
= 3 with a remainder of 1
Again, lets do the same for our third term
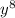
:
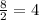
with no remainder, so this term will come out completely.
Finally, lets take our terms out of the radical:
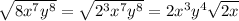
We can conclude that the correct answer is the third one.