Question

A solution is made by mixing 48.0 ml of ethanol, c2h6o, and 52.0 ml of water. assuming ideal behavior, what is the vapor pressure of the solution at 20 °c?

Answer 1

The question is incomplete, here is the complete question:

A solution is made by mixing 45.0 mL of ethanol,
`C_2H_6O`, and 55.0 mL of water. Assuming ideal behavior, what is the vapor pressure of the solution at 20 °C?

Constants @ 20°C:

ethanol = 0.789 g/mL (Density) & 43.9 Torr (Vapor Pressure)

water = 0.998 g/mL (Density) & 17.5 Torr (Vapor Pressure)

Answer: The vapor pressure of the solution at 20°C is 23.4 Torr

Step-by-step explanation:

To calculate the mass of substance, we use the equation:

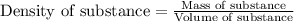`\text{Density of substance}=\frac{\text{Mass of substance}}{\text{Volume of substance}}` ......(1)

To calculate the number of moles, we use the equation:

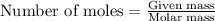`\text{Number of moles}=\frac{\text{Given mass}}{\text{Molar mass}}` .....(2)

Mole fraction of a substance is given by:

`\chi_A=(n_A)/(n_A+n_B)` ......(3)

• For ethanol:

Density of ethanol = 0.789 g/mL

Volume of ethanol = 48.0 mL

Putting values in equation 1, we get:

`0.789g/mL=\frac{\text{Mass of ethanol}}{48.0mL}\\\\\text{Mass of ethanol}=(0.789g/mL* 48.0mL)=37.9g`

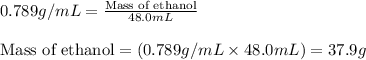
Given mass of ethanol = 37.9 g

Molar mass of ethanol = 46 g/mol

Putting values in equation 2, we get:

`\text{Moles of ethanol}=(37.9g)/(46g/mol)=0.824mol`

• For water:

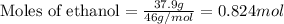
Density of water = 0.998 g/mL

Volume of water = 52.0 mL

Putting values in equation 1, we get:

`0.998g/mL=\frac{\text{Mass of water}}{52.0mL}\\\\\text{Mass of water}=(0.998g/mL* 52.0mL)=51.9g`

Given mass of water = 51.9 g

Molar mass of water = 18 g/mol

Putting values in equation 2, we get:

`\text{Moles of water}=(51.9g)/(18g/mol)=2.88mol`

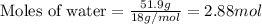
Calculating the mole fractions by using equation 3:

Mole fraction of ethanol,
`\chi_(ethanol)=(n_(ethanol))/(n_(ethanol)+n_(water))=(0.824)/(0.824+2.88)=0.222`

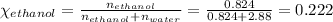
Mole fraction of water,
`\chi_(water)=(n_(water))/(n_(ethanol)+n_(water))=(2.88)/(0.824+2.88)=0.778`

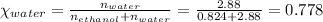
To calculate the total pressure of the mixture of the gases, we use the equation given by Raoult's law, which is:

`p_T=\sum_(i=1)^n(p_(i)* \chi_i)`

`p_T=(p_(ethanol)* \chi_(ethanol))+(p_(water)* \chi_(water))`

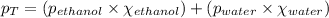
Putting values in above equation, we get:

`p_T=(43.9* 0.222)+(17.5* 0.778)\\\\p_T=23.4Torr`

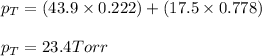
Hence, the vapor pressure of the solution at 20°C is 23.4 Torr

Answer 2

The mass of ethanol = 0.789 g/ml (48) = 37.872 g
Number of moles of ethanol = 37.872 g/ 46g/mol = 0.8233 moles
Mass of water = 52 x 1g/ml = 52 g
Number of moles of water = 52 / 18 g/mol = 2.889 moles of water
We can calculate the mole fraction;
Mole fraction of ethanol = 0.8233/3.712 = 0.2218
Mole fraction of water = 2.889/3.712= 0.7782
But P°(1) Vapor pressure of pure water = 17.5 torr
while P°(2) vapor pressure of pure ethanol = 43.9 torr
P(1) = 0.7782 × 17.5 = 13.6185
P(2) =0.2218 ×43.9 = 9.7370
Therefore, the P(s) = 23.3555