Question

Describe the graph of y=3/4x-12 as compared to the graph of y=1/x

Answer 1

Parent function is compressed by a factor of 3/4 and shifted to right by 3 units.

Explanation:

We are asked to describe the transformation of function
`y=(3)/(4x-12)` as compared to the graph of
`y=(1)/(x)`.

We can write our transformed function as:

`y=(3)/(4(x-3))`

`y=(3)/(4)*(1)/((x-3))`

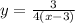
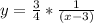
Now let us compare our transformed function with parent function.

Let us see rules of transformation.

`f(x-a)\rightarrow\text{Graph shifted to the right by a units}`,

`f(x+a)\rightarrow\text{Graph shifted to the left by a units}`,

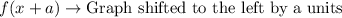Scaling of a function:
`a*f(x)`

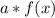
If a>1 , so function is stretched vertically.

If 0<a<1 , so function is compressed vertically.

As our parent function is multiplied by a scale factor of 3/4 and 3/4 is less than 1, so our parent function is compressed vertically by a factor of 3/4.

As 3 is being subtracted from x, so our parent function is shifted to right by 3 units or a horizontal shift to right by 3 units.

Therefore, our parent graph is compressed by a factor of 3/4 and shifted to right by 3 units to get our new graph.