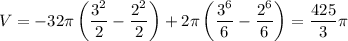You have to use the "Cylinder Method" (aka "Shell Integration").
The volume of the solid obtained by rotating the region between f(x), y=0, x=a, and x=b is given by:
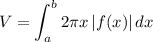
In your case,
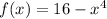
. So, substituiting f(x), a and b, and taking into account that in the interval (2,3) we can assume

, and, therefore,
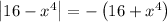
, we get:
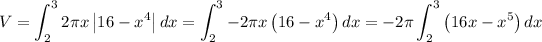
We have made use of the fact that you can take constant factors out of the integral.
Then, being the integral of the sum equal to the sum of the integrals:
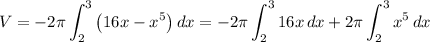
For both integrals we'll use the power integration rule, and the Second Fundamental Theorem of Calculus:
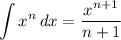
![V= -\displaystyle 2\pi \int^3_2 {16x} \, dx + 2\pi \int^3_2 {x^5} \, dx = -2\pi\cdot16\cdot\left[(x^2)/(2)\right]^3_2 + 2\pi\cdot\left[(x^6)/(6)\right]^3_2](https://img.qammunity.org/2019/formulas/mathematics/high-school/i4p9h7bqx3qx7145mc0tqmzqdyp99vyxfe.png)