Answer: There are no real solutions.
Explanation:
We have the equation:
x = y^2
y = x + 3/2
To solve this, we can start by noticing that x is already isolated in the first equation, then we can replace it in the second equation to get:
y = y^2 + 3/2
And now we can rewrite this as:
y^2 - y + 3/2 = 0
This is a quadratic equation, and the solutions can be calculated with Bhaskara's equation, the solutions are:
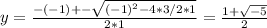
We have a negative number inside the square root, this means that the solutions are complex numbers.
Then we can conclude that the system of equations has no real solutions.