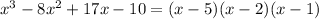Question

Use the x-intercept method to find all real solutions of the equation. x^3-8x^2+17x-10=0

Answer 1

f(2) = 8 - 32 + 34 - 10 = 0

so one real solution is x = 2 and x - 2 is a factor.,

Dividing the function by x -2 gives

x^2 - 6x + 5

x^2 - 6x + 5 = 0

(x - 5)(x - 1) = 0

x = 1 , 5

All real roots are 1,2 and 5.

Answer 2

`x^3-8x^2+17x-10=(x-5)(x-2)(x-1)`

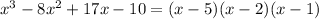
Explanation:

Given : Equation -
`x^3-8x^2+17x-10=0`

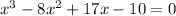
To Find : All real solutions of the equation

Step1 - Write the equation :

`x^3-8x^2+17x-10=0`

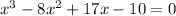
Step 2 : To find the x-intercept we plot the graph,

when we plot the graph the points where y=0 are the x-intercept

Step 3: Points where y=0 are 5,2,1 (shown in the attached graph)

Therefore, (x-5)(x-2)(x-1) are the solutions of the equation.

Hence,
`x^3-8x^2+17x-10=(x-5)(x-2)(x-1)`