Question

Solve for z a(t+z)=45z+67

Answer 1

Solve for z a(t+z)=45z+67
1. Expand

`at+az=45z+67`

2. Subtract
`at` from both sides

`az=45z+67-at`

3. Subtract 45z from both sides

`az-45z=67-at`

4. Factor out the common term z

`z(a-45)=67-at`

5. Divide both sides by a - 45

`z= (67-at)/(ya-45)`

Answer:
`z= (67-at)/(a-45)`

Answer 2

The value of the equation for z is
`z=(at-67)/(45-a)`.

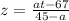Explanation:

Consider the provided equation.

`a(t+z)=45z+67`

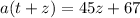
Open the parentheses.

`at+az=45z+67`

Subtract az from both sides.

`at+az-az=45z-az+67`

`at=45z-az+67`

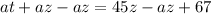
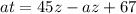
Subtract 67 from both sides.

`at-67=45z-az`

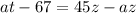
Take z common from right side.

`at-67=z(45-a)`

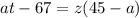
Divide both the sides by 45-a.

`(at-67)/(45-a)=(z(45-a))/(45-a)`

`z=(at-67)/(45-a)`

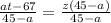
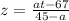
Hence, the value of the equation for z is
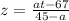`z=(at-67)/(45-a)`.