Answer:
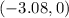 .
.
Explanation:
We have been given that one of the x-intercepts of the parabola
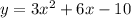 is approximately (1.08, 0). We are asked to find the another x-intercept of parabola.
is approximately (1.08, 0). We are asked to find the another x-intercept of parabola.
We will use quadratic formula to solve our given problem.
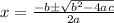
Upon substituting our given values in above formula we will get,
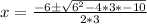

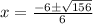
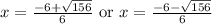

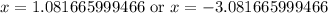
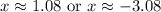
Therefore, the other x-intercept of the parabola is approximately
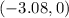 .
.