Question

What is the linear function equation represented by the graph?

Answer 1

`f(x)=(-x)/(2) +1`

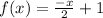
Explanation:

According to the graph, we get the co-ordinates (0,1) and (2,0).

The general form of a linear function is f(x)=mx+c, where m is the slope and c is the y-intercept.

So, using the given co-ordinates (0,1) and (2,0), we will find the slope m.

i.e.
`m=(y_(2)-y_(1))/(x_(2)-x_(1))`

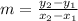
i.e.
`m=(0-1)/(2-0)`

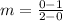
i.e.
`m=(-1)/(2)`

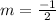
Now, using slope
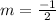`m=(-1)/(2)` and point (0,1), we will find the value of c.

i.e. f(x) = mx + c

i.e. 1 = 0x + c

i.e. c = 1.

Hence, the equation of the graphed linear function is
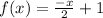`f(x)=(-x)/(2) +1`.