Question

Write Y=3x^2+6x+8 In Vertex form

Answer 1

ANSWER

The vertex form is


`y = 3 {(x + 1)}^(2) + 5`

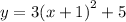
Step-by-step explanation

We want to write


`y = 3 {x}^(2) + 6x + 8`
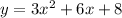
in the vertex form which of the form



`y = a {(x - h)}^(2) + k`

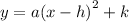
We achieve this BG completing the square.

We proceed as follows,


`y = 3( {x}^(2) + 2x) + 8`


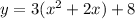
We add and subtract half the coefficient of x multiplied by a factor of 3 which is

`3( (1)/(2) * 2)^(2) = 3( {1)}^(2)`


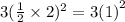
This gives us,



`y = 3( {x}^(2) + 2x) + 3 {(1)}^(2) -3 {(1)}^(2) + 8`

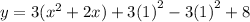
We still factor 3 out of the first two terms to get,


`y = 3( {x}^(2) + 2x + {1}^(2) ) -3 {(1)}^(2) + 8`

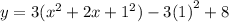
We now got a perfect square, which factors to,



`y = 3 {(x + 1)}^(2) + 5`
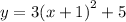
The equation is now in the vertex form.