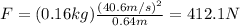Question

In a cricket match, the 0.16kg ball is bowled with a straight arm. During one particular delivery, the ball leaves the bowler's hand horizontally, with a speed relative to the ground of 80.8 mph. The bowler's arm is 0.64 m long and the bowler is running at 10.0 mph when the ball is released.

Calculate the centripetal force exerted on the ball in the bowler's hand, in N.

Note: 1 mph = 0.447 ms^-1.

Answer 1

412.1 N

Step-by-step explanation:

First of all let's calculate the total speed of the ball as it is released, which is equal to the speed of the ball + the speed of the bowler:

`v=80.8 mph +10.0 mph=90.8 mph`

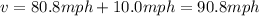
Now let's convert it into m/s. We know that

`1 mph = 0.447 m/s`

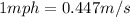
So

`1 mph:0.447 m/s = 90.8 mph:v\\v=((0.447 m/s)(90.8 mph))/(1 mph)=40.6 m/s`

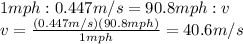
And now we can calculate the centripetal force, which is given by:

`F=m(v^2)/(r)`

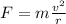
where

m = 0.16 kg is the mass of the ball

v = 40.6 m/s is the speed of the ball

r = 0.64 m is the radius of the circular path (equal to the length of the bowler's arm)

Substituting:

`F=(0.16 kg)((40.6 m/s)^2)/(0.64 m)=412.1 N`