Answer:
Distance covered by hula hoop in 44 rotations = 9671 cm
Explanation:
In the given question,
radius of the hula hoop (r) = 35 cm
and full rotation = 44
Now,
circumference of the hula hoop = 2
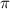 r
r
Then,
distance covered in one rotation = circumference of the hula hoop
distance covered in 44 rotations = 44 × circumference of the hula hoop
Distance = 44 × 2
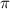 r = 44 × 2 × 3.14 × 35 = 9671.2 cm
r = 44 × 2 × 3.14 × 35 = 9671.2 cm
Distance covered by hula hoop in 44 rotations = 9671 cm