Question

What is the apparent solution to the system of equations? y=−x+2y=4x+7 Graph the system of equations using the Line tool. Plot a point at the apparent solution to the system using the Point tool

Answer 1

To find the apparent solution to the system of equations, you need to solve the equations simultaneously. The apparent solution to the given system of equations is (-1, 3).

Step-by-step explanation:

To find the apparent solution to the system of equations, we need to solve the equations simultaneously. The given equations are:

y = -x + 2

y = 4x + 7

We can solve these equations by setting them equal to each other:

-x + 2 = 4x + 7

Now, we can solve for x:

-x - 4x = 7 - 2

-5x = 5

x = -1

Substituting this value of x back into either of the original equations, we can find the corresponding value of y:

y = -(-1) + 2

y = 3

Therefore, the apparent solution to the system of equations is (-1, 3).

Answer 2

Given the system of equation:

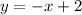`y = -x + 2` and

`y =4x+7`

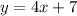
Table for the equation
`y = -x + 2` ;

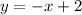x y

-1 -3

0 2

1 1

2 0

Then, the points for this equations are: (-1, -3), (0, 2) , (1, 1 ) and (2, 0)

Also, table for the equation:
`y =4x+7`

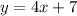
x y

-1.75 0

-1 3

0 7

1 11

Then, the points for this equations are: (-1.75, 0) , (-1, 3) , (0, 7) and ( 1, 11)

Using Point tool , we plot these points of the given system of equation on the Cartesian plane.

You can see the graph as shown below in the attachment.

Also, the two lines intersects at (-1, 3)

Therefore, the apparent solution for the given system of equation is; (-1, 3)