Answer:
1/4
Explanation:
We are to find the scale factor of the dilation that maps the pre-image of triangle ABC with vertices A(2, 5), B(6, 10) and C(9, −1) to the image triangle A'B'C' with vertices A' (0.5, 1.25), B' (1.5, 2.5), C' (2.25, −0.25).
Center of dilation is at the origin.
To find the scale factor, we will divide the corresponding vertices of the image and pre-image.
A(2, 5) ---> A' (0.5, 1.25) =
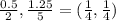
B(6, 10) ---> B' (1.5, 2.5) =
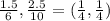
C(9, −1) ---> C' (2.25, −0.25) =
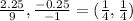
Therefore, the scale factor of the dilation is 1/4.