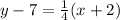Question

Identify an equation in point-slope form for the line perpendicular to y = –4x – 1 that passes through (–2, 7).

Answer 1

Slope of a line perpendicular to an equation is the negative reciprocal of the original equations slope.

In this case the slope is -4 so the line perpendicular will be +1/4

y = (1/4)x + b
7 = (1/4)*-2 + b
7 = -1/2 + b
b = 7 1/2 or 7.5

Full equation is y = (1/4)x + 7.5

Answer 2

Slope-intercept form:

y = mx + b "m" is the slope, "b" is the y-intercept (it's relevant later)

Point-slope form:

y - y₁ = m(x - x₁) "m" is the slope

For lines to be perpendicular, their slopes have to be the opposite/negative reciprocal (flipped sign and number)

For example:

slope is 2

perpendicular line's slope is -1/2

slope is -4/5

perpendicular line's slope is 5/4

Since the given line's slope is -4, the perpendicular line's slope is 1/4.

m = 1/4

(x₁ , y₁) = (-2, 7)

Now plug this into the equation:

y - y₁ = m(x - x₁)

y - 7 = 1/4(x - (-2))

`y - 7=(1)/(4)(x+2)`