Question

Classify the sequence {a_n}={4,10/3,8/3,2...} as arithmetic or geometric. Then, determine whether the sequence is convergent or divergent.

Answer 1

Arithmetic and divergent sequence.

Explanation:

Answer 2

Arithmetic and divergent sequence.

Explanation:

We have been given a sequence:

`a_n=4,(10)/(3),(8)/(3),2...`

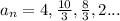
Arithmetic sequence: It is the sequence in which the difference (known as common difference) between consecutive terms is same

Formula for difference:
`d=a_n-a_(n-1)`

Geometric sequence: It is the sequence in which the ratio (known as common ration) between consecutive terms is same.

Formula for ratio:
`(a_n)/(a_(n-1))`

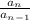
Here, we will first check the common difference

`d=(10)/(3)-4=(-2)/(3)`

`(8)/(3)-(10)/(3)=(-2)/(3)`

`2-(8)/(3)=(-2)/(3)`

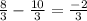
Hence, we are getting common difference therefore it is an arithmetic sequence.

Arithmetic sequence always diverges

And given sequence being arithmetic will diverge.