Answer:
The length of the string is 32.56 feet (Approx) .
Explanation:
As given
A kite is 37 feet in the air and string forms an angle of 62 degrees .
Now by using the trignometric identity.

As shown in the figure given below.
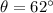
Perpendicular = AB
Hypotenuse = AC = 37 feet
Put in the identity .
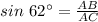
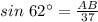
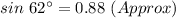
Put in the identity
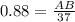
AB = 0.88 × 37
AB = 32.56 feet (Approx)
Therefore the length of the string is 32.56 feet (Approx) .