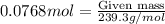Answer: 18.42 grams of lead (II) sulfide will be produced in the given reaction:
Explanation: The reaction of lead (II) acetate and hydrogen sulfide follows:
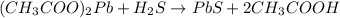
To calculate the moles, we use the formula:
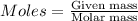 ....(1)
....(1)
Molar mass of lead (II) acetate = 325.29 g/mol
Given mass of lead (II) acetate = 25 g
Putting values in above equation, we get:

We are given that hydrogen sulfide is present in excess, so limiting reagent is lead (II) acetate because it limits the formation of product.
By stoichiometry of the reaction,
1 moles of lead (II) acetate produces 1 mole of lead (II) sulfide
So, 0.0768 moles of lead (II) acetate will produce =
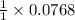 = 0.0768 moles of lead (II) sulfide.
= 0.0768 moles of lead (II) sulfide.
Now, to calculate the mass of lead (II) sulfide, we use equation 1, we get:
Molar mass of lead (II) sulfide = 239.3 g/mol