Answer with explanation:
Distance with time
1. If we plot time on y axis and Distance on x axis ,the set of points will be (0.19,1),(0.38,2),(0.57,3),(0.76,4),(0.95,5),(1.14,6).
Finding the slope between points
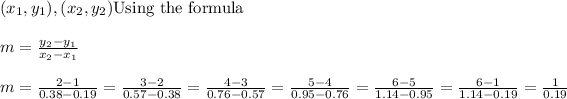
Since the slope between any two points is same ,so the given function is linear.
Elevation with Time
2. If we plot time on y axis and Elevation on x axis ,the set of points will be, (12,1),(26,2),(67,3),(98,4),(124,5),(145,6)
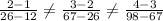
Slope between two points is not same .So, this is not a linear function.
Option B: The elevation is a nonlinear function because it does not have a constant rate of change.