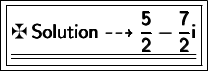
Answer:
Explanation:
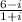
Multiply the numerator & denominstor by the conjugate of 1 - i.
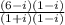
We can solve the denominator by using the identity: a² - b² = (a + b) (a - b). So,
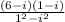
We know that, i² = - 1. Then, on solving..
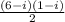
Now, simplify this expression,
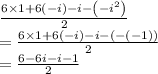
Combine the real & imaginary parts in 6 - 6i - i - 1.
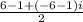
Simplify it again by adding.
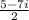
Now seperate the fraction by dividing 5 & -7i by 2.

__________
