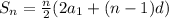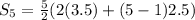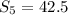ANSWER
Step-by-step explanation
The general term of the arithmetic sequence is
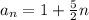
For the first term,we substitute
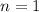
to get,
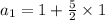
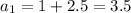
Similarly
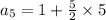
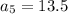
The sum of the first n-terms is given by the formula,
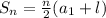
where l is the last term.
In this case
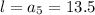
We substitute these values to get,
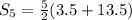
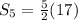
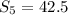
We could have also used the formula,