Question

Solve x+2/x-4<0

A)–4 < x < –2
B)–2 < x < 4
C)–2 < x < –4

Answer 1

-2 < x < 4

Explanation:

Answer 2

`Domain:\ x\\eq-2\\\\(x+2)/(x-4)<0\iff(x+2)(x-4) < 0\\\\x+2=0\to x=-2\\x-4=0\to x=4\\\\(x+2)(x-4)=x^2-4x+2x-8\\\\\text{parabola op}\text{en up}\\\\\text{Look at the picture}\\\\Answer:\ (x+2)/(x-4)<0\ for\ x\in(-2,\ 4)\to\boxed{B)\ -2 < x < 4}`

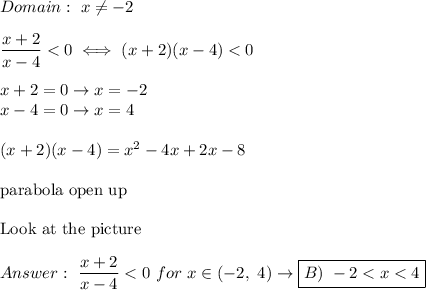
Other method:

`(x+2)/(x-4)<0`

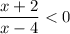
zeros of numerator and denominator are x = -2 and x = 4.

Look at the second picture.

for x < -2 → x + 2 < 0 and x - 4 < 0

therefore
`(x + 2)/(x - 4)=((-))/((-))>0`

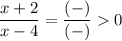
for -2 < x < 4 → x + 2 > 0 and x - 4 < 0

therefore
`(x+2)/(x-4)=((+))/((-)) < 0`

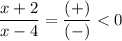
for x > 4 → x + 2 > 0 and x - 4 > 0

therefore
`(x+2)/(x-4)=((+))/((+)) > 0`

Answer: B) -2 < x < 4