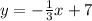Answer:
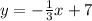
Explanation:
The slope-intercept form is:
 ; where m is the slope, and b is the y-axis interception.
; where m is the slope, and b is the y-axis interception.
So, the problem is asking for a line that it's perpendicular to
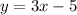 . This perpendicular relation means that the line we have to find have an inverse and opposite slope than the given line, that's expressed like this:
. This perpendicular relation means that the line we have to find have an inverse and opposite slope than the given line, that's expressed like this:
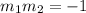
That expression is the condition to have perpendicular lines. So, the given line has a slope of 3, now we can find the slope of the new line:
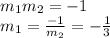
Now we have the slope of the new perpendicular line, we use the point-slope formula to find its equation:
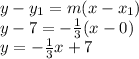
Therefore, the slope-intercept form of the new perpendicular line is: