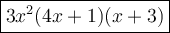Question

Which is the completely factored form of 12x4 + 39x3 + 9x2?

Answer 1

Step-by-step explanation

• Given expression

`{12x}^(4) + {39x}^(3) + {9x}^(2)`

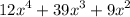
• Factor the expression by using common factor.

We csn pull out 3x² out of the expression.

`{3x}^(2) (4 {x}^(2) + 13x + 3)`

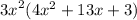
We simply pull out the x-term with lowest degree and 3 out of the expression. If another expression cannot be factored after first factoring then that's the final answer.

• The expression can still be factored. Factor the expression by using two brackets.

`{4x}^(2) + 13x + 3 \\ (4x + 1)(x + 3)`

• New factored form

`3 {x}^(2) (4x + 1)(x + 3)`

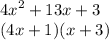
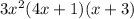
Answer

`\large \boxed { {3x}^(2) (4x + 1)(x + 3)}`