Answer:
The other two factors are (x-2) and (x+4)
Explanation:
Given one root of polynomial
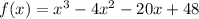 which is x=6
which is x=6
we have to find the other factors of given function.
Polynomial:
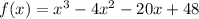
If x=6 is one of the root then by remainder theorem
Put x=6
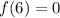
Hence, by synthetic division the quotient is
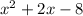
By middle-term splitting method
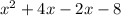

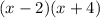
The other two factors are (x-2) and (x+4)