Question

Calculate the number of moles of aluminum, sulfur, and oxygen atoms in 3.00 molesmoles of aluminum sulfate, Al2(SO4)3Al2(SO4)3. Express the number of moles of AlAl, SS, and OO atoms numerically, separated by commas.

Answer 1

`n_(Al)=6.00molAl\\\\n_(S)=9.00molS\\\\n_(O)=36.0molO`

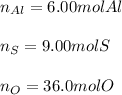
Step-by-step explanation:

Hello!

In this case, when analyzing the moles of an element inside a compound, we need to keep in mind that a mole ratio should be set up; thus, for aluminum sulfate, we have the following ones:

`(2molAl)/(1molAl_2(SO_4)_3) \\\\(3molS)/(1molAl_2(SO_4)_3) \\\\(12molO)/(1mol1molAl_2(SO_4)_3)`

Thus, starting by 3.00 moles of aluminum sulfate, the moles of each element turn out:

`n_(Al)=3.00molAl_2(SO_4)_3*(2molAl)/(1molAl_2(SO_4)_3) =6.00molAl\\\\n_(S)=3.00molAl_2(SO_4)_3*(3molS)/(1molAl_2(SO_4)_3) =9.00molS\\\\n_(O)=3.00molAl_2(SO_4)_3*(12molO)/(1molAl_2(SO_4)_3)=36.0molO`

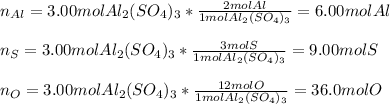
Best regards!