Given:
The sequence 6, 18, 54, 162, …
To Find:
- The common ratio in this sequence
- and the number of pushups Kendall will do on the 20th week.
Answer:
Kendall will do 6973568802 pushups in the 20th week.
Explanation:
The given sequence 6, 18, 54, 162, ... is a geometric series.
A geometric series is a sequence of numbers where each succeeding term can be found by multiplying the previous term with a constant factor which is called the common ratio.
We see that in the given sequence, each successive term can be found by multiplying the previous term by 3.
18 = 6 multiplied by 3
54 = 18 multiplied by 3
162 = 54 multiplied by 3
and so on.
So, the common ratio is 3.
The general form of a geometric series can be written as
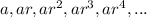
where a denotes the intial term and r denotes the common ratio.
The nth term of the series can be found by the formula
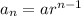
For the given sequence, intial term a = 6 and common ratio r = 3.
To find the number of pushups Kendall will do on the 20th week, we need to calculate the 20th term.
That is,
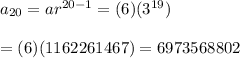
Thus, Kendall will do 6973568802 pushups in the 20th week.