Answer:
The exponential model for the value of the home is
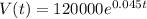 .
.
Explanation:
According to the give information 2002 is the initial year and the value of the hom in 2002 is $120,000.
The model will have the form
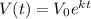
Where V₀ is initial value of home, k is a constant and t is number f years after 2002.
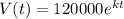
The value of home in 2007 is $150,000. Difference between 2007 and 2002 is 5 years. Therefore the value of function is 150000 at t=5.
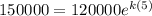
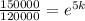
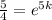
Take ln both sides.
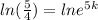
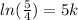 (
(
 )
)
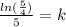
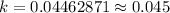
Therefore exponential model for the value of the home is
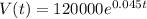 .
.
Where t is number of years after 2002.