Question

A sleeping 68 kg man has a metabolic power of 71 W . How many calories does he burn in 8 hours of sleep?

Answer 1

`4.87\cdot 10^5 cal`

Step-by-step explanation:

Power is related to energy by the formula:

`P=(E)/(t)`

where

P is the power

E is the energy used

t is the time taken

In this problem, we know the power, P = 71 W, and the time taken:

`t=8 h \cdot 3600 =28800 s`

so, the energy burned is

`E=Pt=(71 W)(28800 s)=2.04\cdot 10^6 J`

And since 1 cal = 4.186 J, we can convert into calories

`E=(2.04\cdot 10^6 J)/(4.186)=4.87\cdot 10^5 cal`

Answer 2

Energy, E = 488.71 calorie

Step-by-step explanation:

It is given that,

Mass of the man, m = 68 kg

Metabolic power of the man, P = 71 W

Time taken, t = 8 hours = 28800 s

Let E is the amount of energy transferred in given time. It can be calculated as :

`E=P* t`

`E=71\ W* 28800\ s`

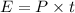
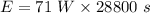
E = 2044800 J

or

E = 2044.8 kJ

Since,

1 calorie = 4.19 kJ

E = 488.71 calorie

So, he burn 488.71 calorie in 8 hours of sleep. Hence, this is the required solution.