Question

Suppose you hike 3 miles from the campgrounds to the lake at a rate of x miles per hour. On your way back from the lake to the campgrounds, your rate was 1 mile per hour faster. If it took you 2.5 hours for the complete round trip, which equation could be used to determine your rate?

Answer 1

Answer: The following three equations could be used to determine the rate:

x*(time there) = 3 miles

(x+1)*(time back) = 3 miles

(time there) + (time back) = 2.5 hr

Step by step (including the actual solution x):

Equation for the trip there:

x*(time there) = 3 miles

Equation for the trip back:

(x+1)*(time back) = 3 miles

And we also know that:

(time there) + (time back) = 2.5 hr

So we have three equations with three unknowns and can solve for x. Let us call

(time there): t1

(time back): t2

`x\cdot t_1 = 3\\(x+1)\cdot t_2 = 3\\t_1 + t_2 = 2.5\implies t_1 = 2.5-t_2\\\\x(2.5-t_2) = 3\\(x+1)\cdot t_2 = 3\\\\...\implies x = 2, t_1=1.5, t_2=1\\`

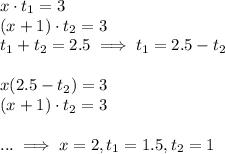
The solution (I skipped the gory details of solving, but you can verify by plugging the values back into the equations) is

Rate x = 2 miles/hour

(time there) = 1.5 hours

(time back) = 1 hour

Answer 2

the correct answer is c

Explanation: