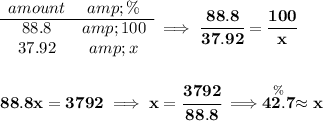we'll proceed along the same lines as the previous one.
the cost of the boots is 90.10 and that includes 6.25% sales tax, so if "x" is the 100%, then 90.10 is really the 106.25%, because is including the extra 6.25%.
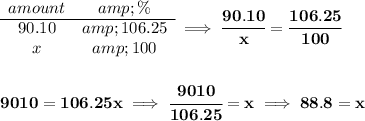
so if that's the cost of each boot, without tax, then the markup will be 88.8 - 50.88 = 37.92.
if the 100% is 88.8, how much is 37.92 off of it in percentage?