Question

I need help please.

1. Write a function defined by y = ƒ(x), subject to the condition that the value of ƒ(x) is 16 times the square root of x.

4. Which of the following is a solution of 4x + 2y ≤ 6?.

5. Solve the system using the addition method.
–5x – y = 38
–6x – 3y = 60

6. If ƒ(x) = 15x + 7 and g(x) = x2 – 5x, find (ƒ + g)(x).

7. Which one of the following points is a solution to the system shown? -8x-8y-5z=-6
7x-8y-9z=17
9x+2y+6z=-1

8. Graph the solution set of the inequality: 5x ≤ 7

9. Find the domain of the function shown. Write your answer in interval notation.
g(y) = √ y – 6

10. The value y is a function of x if for each element x in the domain, there is/are how many value(s) of y in the range?

Answer 1

1. It is given that f(x) is 16 times the square root of x.

Putting that in mathematical terms we have,

f(x) = 16 *
`√(x)`

also, y = f(x)

So, we have the function

y= 16
`√(x)`

4. We need to solve the inequality equation : 4x + 2y ≤ 6

Let us take the equation,

4x+2y ≤ 6

2y ≤ 6-4x

y ≤
`(6-4x)/(2)`

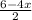
y ≤ 3-2x

So, any point (x,y) lies on the given inequality region, where y≤ 3-2x

5. Solving system of equations using addition method

Given:

-5x-y =38 ------> a

-6x-3y =60 -------> b

Divide the equation b by no. 3

(-6x-3y)/3 = 60/3

-2x-y = 20 -------> c

Subtracting equation c from a we have,

-5x-y - (-2x-y) = 38 - 20

-5x-y+2x+y = 18

-3x = 18

x = -6

Now, substituting the value of x in equation a, we get

-5(-6) - y =38

30-y =38

y= 30-38 = -8

y=-8

∴ x = -6 and y = -8

6. Finding composition of functions

Given : f(x) =15x + 7 ; g(x) = x² - 5x

To find : (f+g)(x)

(f+g) (x) = f(g(x))

So, replace the value of x in f(x) by g(x), where g(x)= x² - 5x

(f+g)(x) = 15(x²-5x) +7 =15x²-75x+7

∴ (f + g)(x) = 15x²-75x+7

7. System of 3 equations must be solved to find the solution

-8x-8y-5z=-6

7x-8y-9z =17

9x+2y+6z =-1

Solving by substitution method.

Isolate x from first equation :

x= (-6+8y+5z)/(-8)

Substitute this value of x in 2nd and 3rd equations.

`7 (- (-6+8y+5z)/(8))-8y -9z = 17`

`9(- (-6+8y+5z)/(8)) + 2y + 6z = -1`

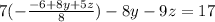
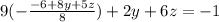
Now, isolating y from the 2nd equation rewritten above, we have

y= -
`(107 z + 94)/(120)`

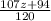
Now substituting this value of y in the 3rd equation rewritten above, we have

`9(- (-6+8(-(107 z + 94)/(120))+5z)/(8)) + 2(-(107 z + 94)/(120)) + 6z = -1`

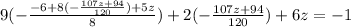
Isolating z from above equation, we have

z = -2

Substitute z= -2 in the equation of y, we have

y= -
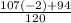`(107 (-2) + 94)/(120)` = 1

y = 1

Substituting the value of y and z, in the equation of x, we have

x= (-6+8(1)+5(-2))/(-8) = 1

x = 1

∴ x=1 ; y = 1 ; z = -2

8. 5x ≤ 7

Solving the above equation, we have

x ≤ 7/5

Please see attachment for the graph.

9. The given function is : g(y) =
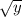`√(y)` -6

The domain is the set of values of y for which there can be a value of g(y).

Here g(y) can be real only if y is greater than or equal to 0.

∴ The domain of the given function is [0,∞) .

10. Given : y is a function of x.

Definition of function : A function is a relation that associates each element in the domain to one element of another set, the co-domain of the function.

∴ For each element x, in the domain, there is only one value of y in the range.