Question

An aeroplane takes off and flies steadily for a distance of 5.6 km at an altitude of 2 km from it's runway . Calculate the angle that it makes with the horizontal

Answer 1

The angle the aero plane makes with the horizontal is approximately 20.925°

Explanation:

The given parameters are;

The distance the aero plane flies = 5.6 km

The elevation to which the aero plane flies = 2 km

The angle the path of the aero plane as it takes off makes with the horizontal, θ, is the angle opposite the altitude of the right triangle formed by the aero plane's path (the hypotenuse), the horizontal, and the line drawn from the final position of the aero plane, representing the altitude (the opposite leg to angle θ)

By the trigonometric ratio for the sin of the angle, θ, we have;

`sin(\theta) = (Opposite \ leg \ length)/(Hypotenuse \ length) = (2 \ km)/(5.6 \ km) = (2)/(5.6)`

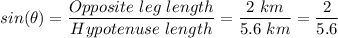
Therefore, the angle θ the aero plane makes with the horizontal is given as follows;

`\theta = arcsin \left ((2)/(5.6) \right ) \approx 20.925 ^(\circ)`

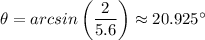
The angle the aero plane makes with the horizontal ≈ 20.925°.