Question

Area of triangle- please help :)

Answer 1

I will help you as soon as possible

Answer 2

`\approx 45.1\:\mathrm{w/\: some \: MOE}`

Explanation:

The area of
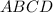`ABCD` is comprised of two triangles, so we can find the area of both triangles and add them to get the total area of

Since the sum of the interior angles of a triangle add up to
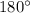`180^(\circ)`, we have:

`\angle C=180-44-38=\fbox{$98^(\circ)$}`.

The Law of Sines is given as:

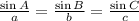`(\sin A)/(a)=(\sin B)/(b)=(\sin C)/(c)` for any triangle.

Therefore, we have the proportion:

`(\sin 98^(\circ))/(10)=(\sin 44^(\circ))/(BC),\\BC=(10\cdot \sin 44^(\circ))/(\sin 98^(\circ)),\\BC\approx 7.01`.

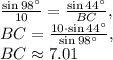Now that we have two sides of a triangle and the angle between them, we can use
`[\triangle]=(1)/(2)ab\sin C` to find the area of
`\triangle CBD`:

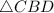`[CBD]=(1)/(2)\cdot 10\cdot 7.01 \cdot \sin 38^(\circ),\\\\\left [CBD]\right\approx 21.58`.

Since
`\triangle ABD` is a right triangle, we can use basic trig rules for a right triangle to solve for the sides:

`\sin 55^(\circ)=(AB)/(10),\\AB=10\sin 55^(\circ),\\AB\approx 8.19`,

`\sin 35^(\circ)=(AD)/(10),\\AD=10\sin 35^(\circ),\\AD\approx 5.74`.

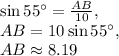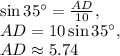Therefore, the area of
`\triangle ABD` is:

`(1)/(2)\cdot 8.19\cdot 5.74\approx 23.49`.

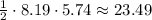The area of
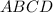`ABCD` is then

`21.58+23.49=45.07=\fbox{$45.1$}`.

`\bigstar` Note that intermediate results were rounded during this calculation. Therefore, there is a small margin of error when rounding to final answer to one decimal place. I recommend plugging in values for yourself using all equations I wrote above, then rounding only your final answer to one decimal place as requested by the problem.