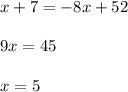Given:
The equation
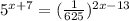
Answer:
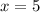
Explanation:
To simplify the given expression, we use the Laws of Exponents; that is, when the bases are the same in two sides of the equation, the exponents can be equated.
So, we begin by expressing 625 on the right hand side as a power of 5 which is the same base as on the left hand side.
Then, we equate the exponents and simplify for x as we usually do.
The following is the solution.
We know

and
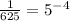
So,
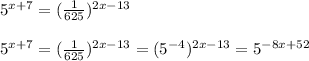
We now equate the exponents on both sides as solve for x like we would normally do