Consider rectangular box with
- length x units (x≥0);
- width 3 units;
- height (8-x) units (8-x≥0, then x≤8).
The volume of the rectangular box can be calculated as
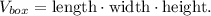
In your case,
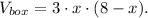
Note that maximal possible value of the height can be 8 units (when x=0 - minimal possible length) and the minimal possible height can be 0 units (when x=8 - maximal possible length).
From the attached graph you can see that the greatest x-intercept is x=8, then the height will be minimal and lenght will be maximal.
Then the volume will be V=0 (minimal).
Answer: correct choices are B (the maximum possible length), C (the minimum possible height)