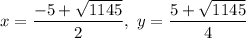x, y - two positive numbers
one positive number is 5 less than twice a second number
(1) x = 2y - 5
their product is 140
(2) xy = 140
substitute from (1) to (2)
(2y - 5)y = 140 use distributive property
(2y)(y) - (5)(y) = 140
2y² - 5y = 140 subtract 140 from both sides
2y² - 5y - 140 = 0
use quadratic formula
a = 2, b = -5, c = -140
b² - 4ac = (-5)² - 4(2)(-140) = 25 + 1120 = 1145
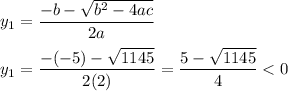
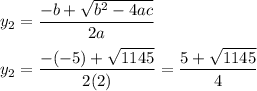
substitute the value of y₁ to (1)
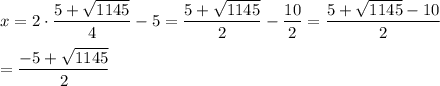
Answer: