Question

Two rival dry cleaners both advertise their prices. Let x equal the number of items dry cleaned. Store A's prices are represented by the expression 15x - 2. Store B's prices are represented by the expression 3(5x + 7). When do the two stores charge the same rate?

Answer 1

Answer::

Givens

Store A's price is
`15x-2`

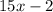
Store B's price is
`3(5x+7)`

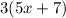
Where
`x` represents the number of items.

Now, observe that the coefficient of both expressions is 15, because

`3(5x+7)=15x+21`

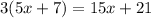
Remeber that the coefficient of the x-variable is always the constant rate of change. Also, the given expressions can be expressed as linear functions

`y=15x-2\\y=15x+21`

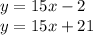
Observe that both Stores have already the same rate, which is 15.

Therefore, they have the same rate at any number of items dry cleaned.

What they have different is their initial condition, -2 and 21 respectively, but the rate is the same.

Answer 2

Store A's prices are represented by the expression 15x - 2.

Store B's prices are represented by the expression 3(5x + 7).

To find when the 2 stores charge the same rate , we set the expression equal and solve for x

15x - 2 = 3(5x+7)

15x -2 = 15x + 21

Subtract 15x on both sides

-2 = 21

We cannot solve for x

So , the price of two stores never be same

Two stores never charge the same rate